Perimeter of polygons with an inscribed circle
Key Notes :
A tangent to a circle is a line that intersects a circle at exactly one point. The point where the line intersects the circle is called the point of tangency.
If a line is tangent to a circle, then the line is perpendicular to the radius at the point of tangency.
For example, BC is tangent to ⨀A at point B. So, BC is perpendicular to radius AB at point B.
The converse is also true. If a line is perpendicular to the radius of a circle at a point on the circle, then the line is tangent to the circle.
Solving problems involving tangents
You can use what you know about tangents to solve problems.
Determining if a line is tangent to a circle
Let’s try it! Determine if KL is tangent to ⨀M.
If KL is perpendicular to the radius KM, then KL is tangent to ⨀M. So, check whether ∠K is a right angle. The converse of the Pythagorean theorem states that △KLM is a right triangle with hypotenuse LM if KL2+KM2=LM2.
KL2+KM2 ≟ LM2
82+152≟172
64+225≟289 Square.
289=289 Add.
Since KL2+KM2=LM2 . △KLM is a right triangle with hypotenuse LM . Therefore, ∠K is a right angle, and KL is perpendicular to KM is tangent to ⨀M.
Finding the radius of a circle
ST is tangent to ⨀R. Find the radius of the circle.
Since ST is tangent to ⨀R, ST is perpendicular to RT. So, △RST is a right triangle with hypotenuse RS. You can use the Pythagorean theorem to find the radius of the circle, x.
RT2+ST2=RS2
x2+212=(x+9)2 Plug in RT=x, ST=21, and RS=x+9.
x2+441=x2+18x+81 Square 21 and (x+9).
441=18x+81 Subtract x2 from both sides.
360=18x Subtract 81 from both sides.
20=x Divide both sides by 18.
So, the radius of ⨀R is 20 inches.
Circles with two tangents
If two line segments are tangent to a circle and share a common endpoint outside the circle, then the line segments are congruent.
For example, XW and XY are both tangent to ⨀Z. Those line segments have a common endpoint, X, which lies outside of ⨀Z. So, XW≅XY.
Solving problems with two tangents
Let’s try it! DE and EF are tangent to ⨀G. Find DE.
DE and EF are tangent to ⨀G. Also, DE and EF have a common endpoint, E, that lies outside of circle ⨀G. So, DE≅EF. Set DE equal to EF and solve for x.
DE=EF
2x+3=3x–1 Plug in DE=2x+3 and EF=3x–1
2x+4=3x Add 1 to both sides.
4=x Subtract 2x from both sides.
Now, find DE. Plug in x=4 and solve for DE.
DE=2x+3
=2(4)+3 Plug in x=4.
=11 Simplify.
So, DE=11.
Constructing a tangent to a circle
You can construct a tangent to a circle using a straightedge and a compass.
Tangent that passes through a given point on the circle
To construct the tangent to a circle that passes through a given point on the circle, start with the circle, center, and point on the circle. Call the center point A and the point on the circle point B.
Now, use your straightedge to draw a line segment with endpoint A that passes through point B. Extend the line segment outside of the circle.
Then, construct a line segment that has point B as a midpoint. Set your compass to a setting shorter than AB and place the point of your compass at point B. Draw two arcs using that setting. One of the arcs should intersect the line segment inside of the circle, and the other arc should intersect the line segment outside of the circle. Mark the points where the arcs intersect the line segment. Call them points C and D. You now have CD, which has midpoint B.
The remainder of the steps involve constructing the perpendicular bisector of CD. Set your compass to a setting greater than 1/2CD and place the point of your compass at point C. Draw an arc near CD.
Next, using the same compass setting, place the point of your compass at point D. Draw an arc that intersects the arc you drew in the previous step. Mark the point where the arcs intersect. Call it point E.
Last, use your straightedge to draw a line that connects points B and E.
BE is the perpendicular bisector of CD, so it is perpendicular to radius AB. Thus, BE is tangent to ⨀A at point B.
Tangent that passes through a given point outside of the circle
You can also construct a tangent to a circle that passes through a given point outside of the circle. Start with the circle, center, and point outside of the circle. Call the center point P and the point outside of the circle point Q.
Now, use your straightedge to draw a line segment with endpoints P and Q.
The next few steps involve constructing the perpendicular bisector of PQ. Set your compass to a setting greater than 1/2PQ and place the point of your compass at point P. Draw arcs above and below the line segment.
Next, using the same compass setting, place the point of your compass at point Q. Draw arcs above and below the line segment. Mark the points where the arcs intersect. Call them points R and S.
Then, draw a line that connects points R and S using your straightedge. This is the perpendicular bisector of PQ. Mark the point where RS intersects PQ. Call it point T. RS is the perpendicular bisector of PQ, so point T is the midpoint of PQ.
After that, construct the point where the tangent intersects the circle. Set your compass to a setting equal to PT and place the point of your compass at point T. Draw an arc that intersects the circle. Mark the point where the arc intersects ⨀P, and call it point U.
Last, use your straightedge to draw a line segment that has endpoints Q and U.
QU is tangent to ⨀P at point U.
Why does this construction work?
To understand why QU is tangent to ⨀P, you can draw the entire circle for the arc that intersected ⨀P. This circle is centered at point T. Since ⨀T was created with a radius of PT and point T is the midpoint of PQ, PQ is a diameter of ⨀T.
You can also add PU to create ∠PUQ. Since ∠PUQ is inscribed in a semicircle, it must be a right angle.
So, QU is perpendicular to PU at point U. Notice that PU is a radius of ⨀P. Since QU is perpendicular to a radius of ⨀P at a point on the circle, QU is tangent to ⨀P.
Learn with an example
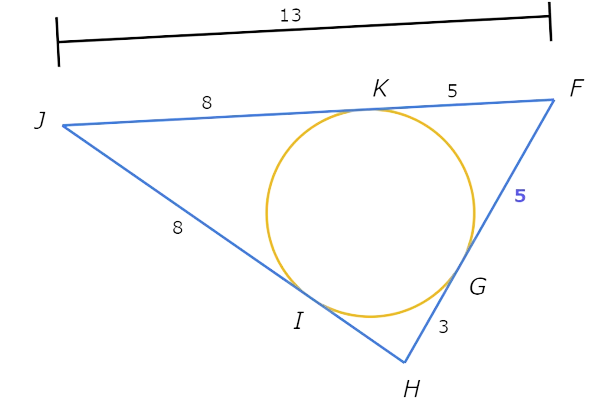
What is FG?
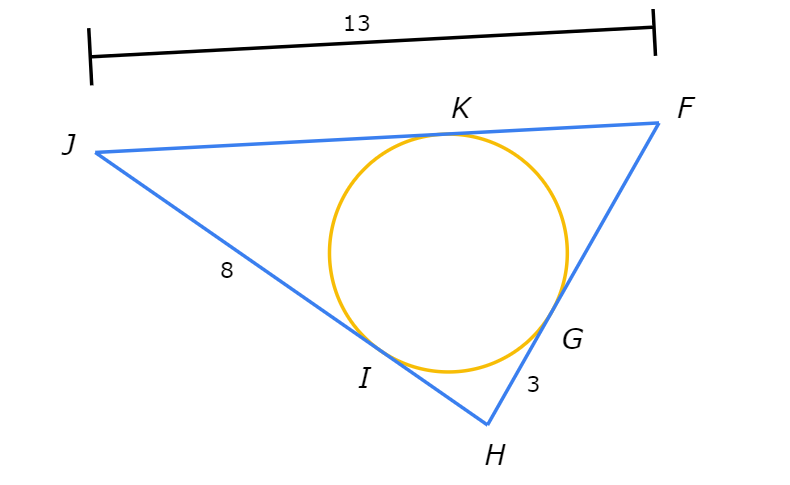
FG= _______
Look at the diagram:
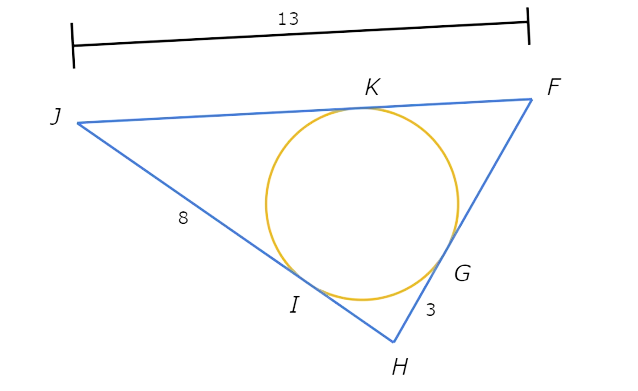
Find the unknown segment lengths.
JK and IJ are tangents to the inscribed circle from J. So , JK is congruent to IJ.
JK=IJ=8.
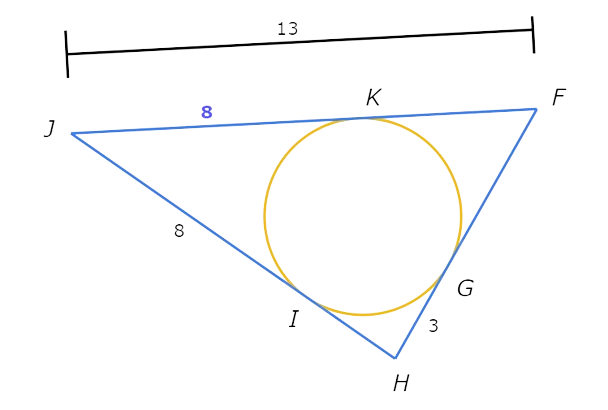
You know JK and FJ. Use the Additive Property of Length to write an equation and find FK.
FK+JK= FJ Additive Property of Length
FK + 8 = 13 Plug in JK=8 and FJ=13
FK = 5 Subtract 8 from both sides
So, FK is 5.
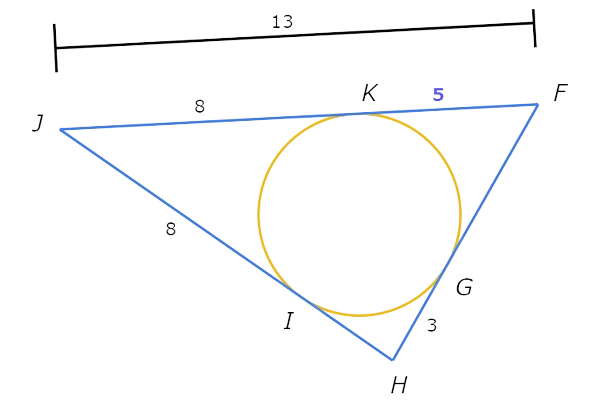
FG and FK are tangents to the inscribed circle from F. So , FG is congruent to FK.
FG=FK=5.
What is TU?
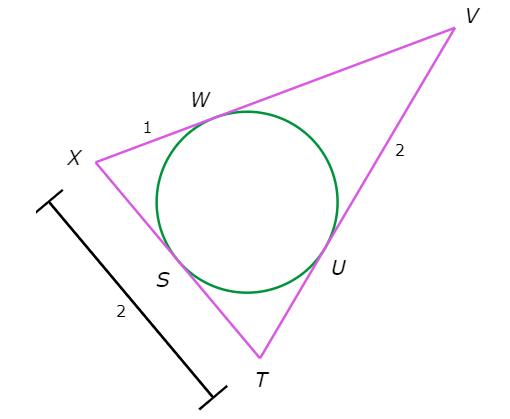
TU=______
Look at the diagram:
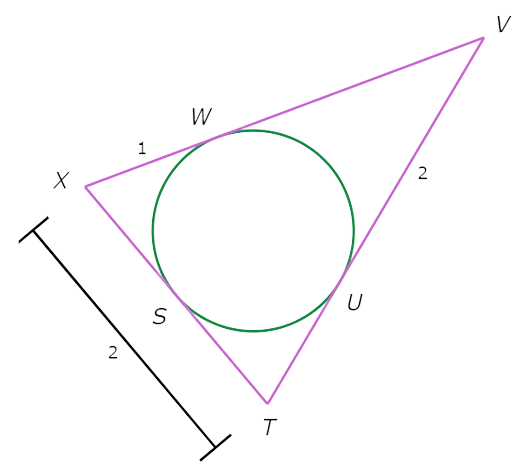
Find the unknown segment lengths.
SX and WX are tangents to the inscribed circle from X. So , SX is congruent to WX .
SX=WX=1.
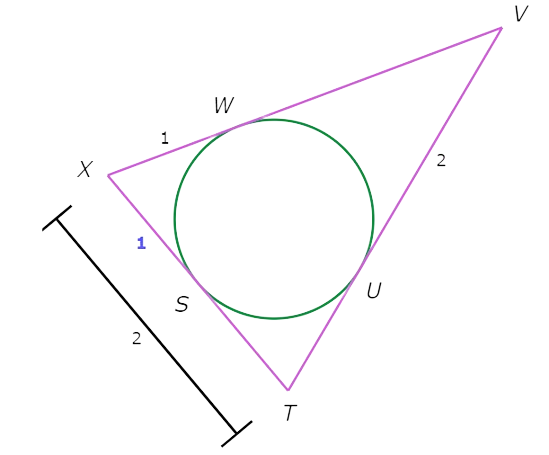
You know SX and TX. Use the Additive Property of Length to write an equation and find ST.
SX + ST = TX Additive Property of Length
1 + ST = 2 Plug in SX=1 and TX=2
ST= 1 Subtract 1 from both sides
So, ST is 1.
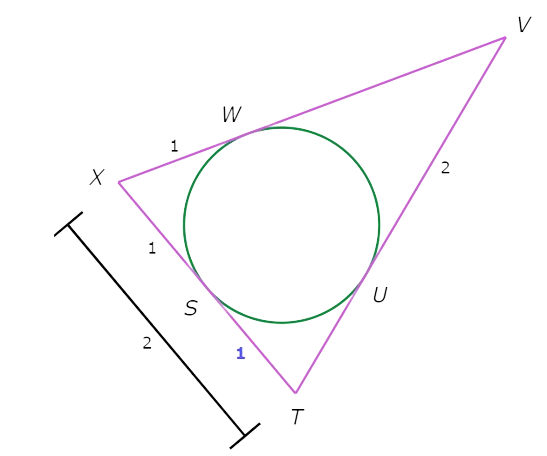
TU and ST are tangents to the inscribed circle from T. So , TU is congruent to ST.
TU=ST=1.
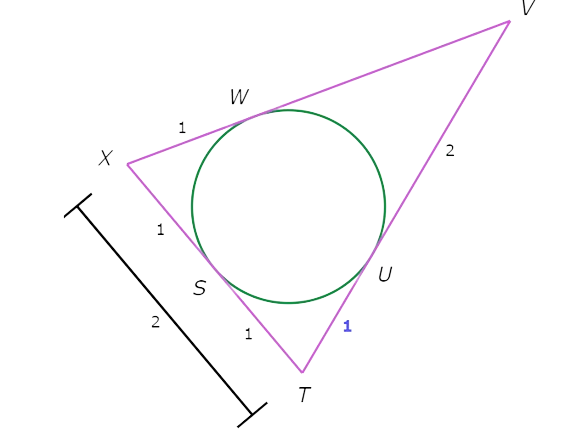
let’s practice!

