Area of sectors

Key Notes :

A sector of a circle is a region bounded by an arc of the circle and the two radii that intersect the arc’s endpoints.

A circle is shown. There is a shaded region of the circle that is bounded by an arc of the circle and the two radii that intersect the arcs endpoints. The shaded region is labeled sector.

Here is a formula for the area of a sector:

K = m / 360 . A

In this formula, K is the area of the sector, m is the degree measure of the arc bounding the sector (or the central angle that intercepts the arc), and A is the area of the circle.

Tip

You can also write this formula as a proportion where each ratio relates the sector to the full circle:

K / A = M / 360

The ratio K / A compares the area of the sector to the area of the circle. The ratio m / 360

compares the degree measure of the arc bounding the sector to the degree measure of a full circle.

Finding area of sectors

Let’s try it! Find the area of shaded sector BCD.

Circle C is shown. Sector BCD, which is bounded by a 72 degree arc, is shaded. Sector BCD is also bounded by two radii, one of which is labeled 5 inches.

You can use the measure of BD and the area of ⨀C to find the area of shaded sector BCD. Find the area of the circle using the formula A = 𝜋r2 , where r is the radius.

A= 𝜋r²

= 𝜋5² Plug in r=5 .

= 25𝜋 Simplify.

The area of the circle is 25𝜋 square inches.

Now, find the area of the sector.

K = M / 360 . A

K = 72 / 360 . 25𝜋 Plug in m=72 and A=25𝜋.

K = 5𝜋 Simplify.

So, the area of shaded sector BCD is 5𝜋 square inches.

Finding arc measures

To find the measure of an arc, you can use the area of the related sector and the area of the circle.

Let’s try it! Find the measure, in degrees, of FIH.

Circle G is shown. Sector FGH, which is bounded by arc FIH and has an area of 80 pi centimeters squared, is shaded. Shaded sector FGH is also bounded by two radii, one of which is labeled 10 centimeters.

FIH is the arc that bounds shaded sector FGH. So, you can use the area of shaded sector FGH and the area of ⨀G to find the measure of the arc. The area of the shaded sector is 80𝜋 square centimeters. To find the area of the circle, you can use the formula A=𝜋r2, where r is the radius.

A=𝜋r²

= 𝜋10² Plug in r=10.

= 100𝜋 Simplify.

The area of the circle is 100𝜋 square centimeters.

Now, find the measure of the arc.

K = M / 360 . A

80𝜋 = M / 360 . 100𝜋 Plug in K=80𝜋 and A=100𝜋 .

28,800𝜋 / 100𝜋 = m Multiply both sides by 360 / 100𝜋.

288= m Simplify.

So, the measure of FIH is 288°.

Area of sectors and radians

You can also find the area of a sector when the arc measure is given in radians. Here is a formula for the area of a sector, where K is the area of the sector, r is the radius of the circle, and 𝜃 is the radian measure of the arc bounding the sector:

K= 1/2 ^r2𝜃

Finding area of sectors

Let’s try it! Find the area of shaded sector XYZ.

Circle Y is shown. Sector XYZ, which is created by a central angle measuring 2 pi over 3 radians, is shaded. Sector XYZ is also bounded by two radii, one of which is labeled 6 meters.

Since you know the measure of ∠XYZ in radians, you can use the radius of ⨀Y and the measure of XZ to find the area of shaded sector XYZ. The measure of XZ is equal to the measure of the central angle that intercepts it. So, the measure of XZ is 2𝜋3 radians.

K= 1/2 ^r2𝜃

= 1/2 . 6² . 2𝜋 / 3 Plug in r=6 and 𝜃= 2𝜋 / 3

=12𝜋 Simplify.

So, the area of shaded sector XYZ is 12𝜋 square meters.

Finding arc measures

To find the measure of an arc in radians, you can use the area of the related sector and the radius of the circle.

Let’s try it! Find the measure, in radians, of SU.

Circle T is shown. Sector STU, which has an area of 6 pi feet squared, is shaded. The diameter of the circle is labeled 8 feet.

SU is the arc that bounds shaded sector STU. So, you can use the area of shaded sector STU and the radius of ⨀T to find the measure of the arc. The area of the shaded sector is 6𝜋 square feet. You’re given the diameter of the circle, 8 feet, which is 2 times the length of the radius. So, the radius of the circle is 4 feet.

K= 1/2 ^r2𝜃

6 𝜋 = 1/2 . 4² . 𝜃 Plug in K=6𝜋 and r=4

6𝜋=8𝜃 Multiply.

6𝜋 / 8 = 𝜃 Divide both sides by 8.

3𝜋 / 4 = 𝜃 Simplify.

So, the measure of SU is 3𝜋 / 4 radians.

Learn with an example

The radius of a circle is 10 centimetres. What is the area of a sector bounded by a 180° arc?

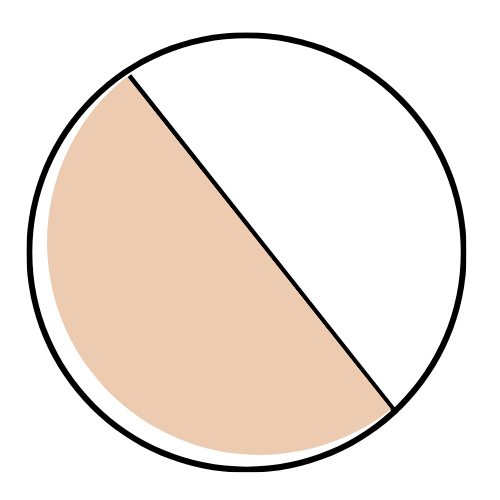
Give the exact answer in simplest form.

___________ square centimetres

The sector’s area depends on the arc’s measure and the circle’s area. You already know that the arc’s measure is 180°, so find the circle’s area.

First, find the area of the circle.

A = 𝜋 r²

= 𝜋 10² Plug in r=10

= 100 𝜋 Square

The area of the circle is 100𝜋 square centimetres.

Now, find the area of the sector.

K = A . m/360

= 100 𝜋 180 / 360 Plug in A=100𝜋 and m=180

= 50 𝜋 Multiply and simplify

The area of the sector is 50𝜋 square centimetres.

The radius of a circle is 8 kilometres. What is the area of a sector bounded by a 45° arc?

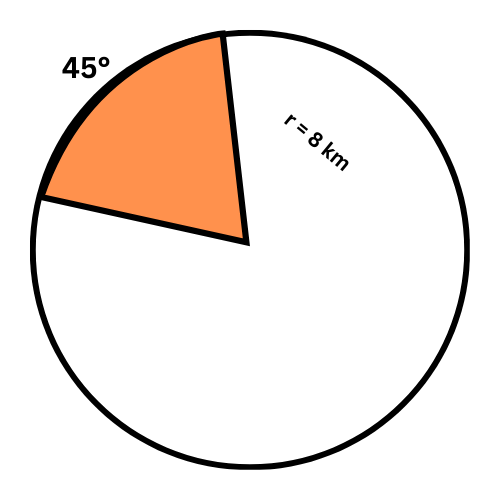
Give the exact answer in simplest form.

_________ square kilometres

The sector’s area depends on the arc’s measure and the circle’s area. You already know that the arc’s measure is 45°, so find the circle’s area.

First, find the area of the circle.

A = 𝜋 r²

= 𝜋 8² Plug in r=8

= 64 𝜋 Square

The area of the circle is 64𝜋 square kilometres.

Now, find the area of the sector.

K = A . m/360

= 64 𝜋 45 / 360 Plug in A=64𝜋 and m=45

= 8 𝜋 Multiply and simplify

The area of the sector is 8𝜋 square kilometres.

The radius of a circle is 6 centimetres. What is the area of a sector bounded by a 90° arc?

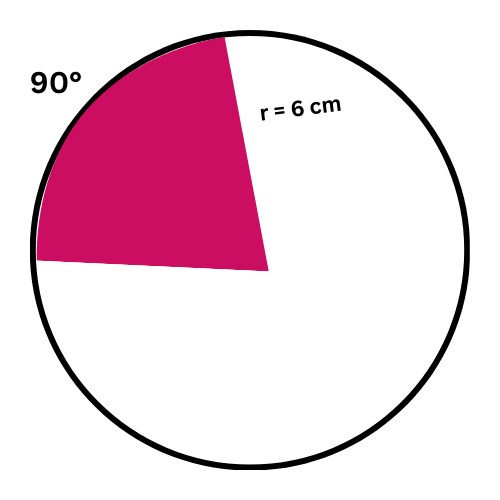
Give the exact answer in simplest form.

___________ square centimetres

The sector’s area depends on the arc’s measure and the circle’s area. You already know that the arc’s measure is 90°, so find the circle’s area.

First, find the area of the circle.

A = 𝜋 r²

= 𝜋 6² Plug in r=6

= 36 𝜋 Square

The area of the circle is 36𝜋 square centimetres.

Now, find the area of the sector.

K = A . m/360

= 36 𝜋 90 / 360 Plug in A=36 𝜋 and m=90

= 9 𝜋 Multiply and simplify

The area of the sector is 9𝜋 square centimetres.

Let’s practice!