Central angles

Key Notes :

The measures of the central angles in a circle add up to 360°.

🔔 What is ∠GJH?

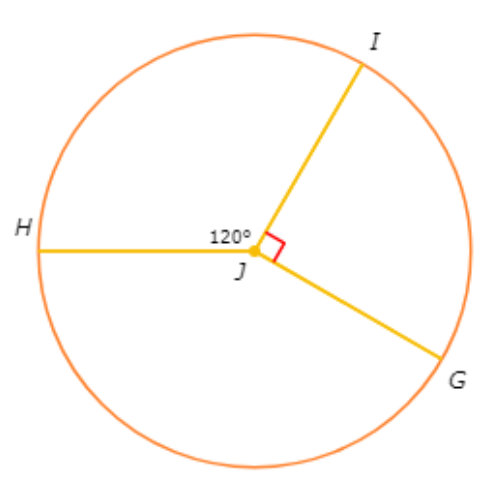
This image has an empty alt attribute; its file name is Untitled-500-%C3%97-500px-1.png

∠GJH= ______ °

Look at the diagram:

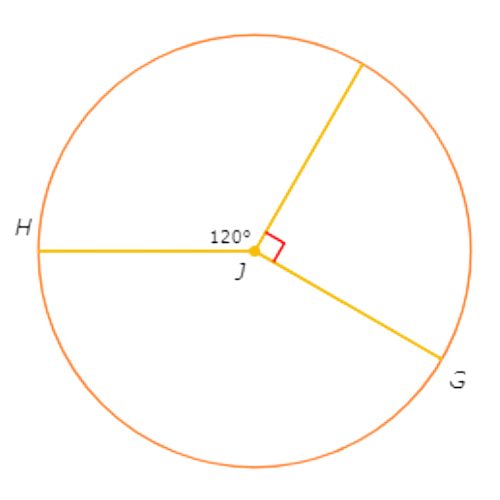
This image has an empty alt attribute; its file name is Untitled__500___500px___1_-removebg-preview.png

Write an equation showing that the measures of ∠GJH, ∠HJI and ∠GJI sum to 360°, since they span an entire circle. Then solve for ∠GJH.

∠GJH + ∠HJI + ∠GJI = 360°

∠GJH+120°+90° = 360° Plug in ∠HJI=120° and ∠GJI=90°

∠GJH+210° = 360° Combine like terms

∠GJH = 150° Subtract 210° from both sides

So, ∠GJH=150°.

🔔 What is ∠QPR?

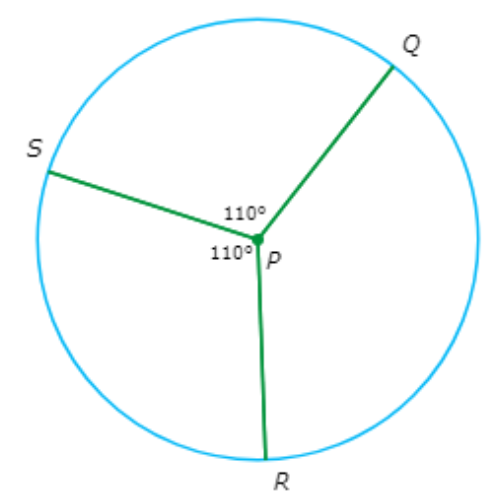
This image has an empty alt attribute; its file name is Untitled-500-%C3%97-500px.png

∠QPR= _____°

Look at the diagram:

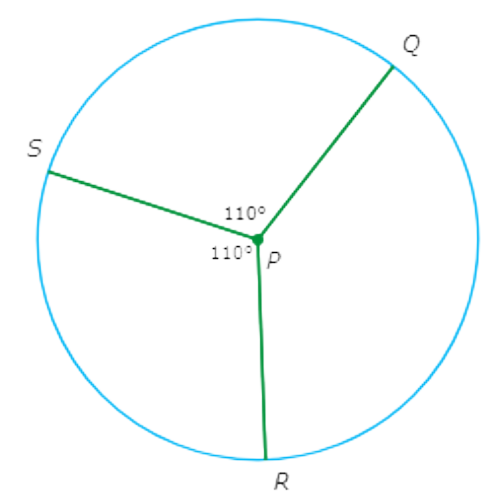
This image has an empty alt attribute; its file name is Untitled__500___500px_-removebg-preview-1.png

Write an equation showing that the measures of ∠RPS, ∠QPS and ∠QPR sum to 360°, since they span an entire circle. Then solve for ∠QPR.

∠RPS+∠QPS+∠QPR = 360°

110°+110°+∠QPR = 360° Plug in ∠RPS=110° and ∠QPS=110°

∠QPR+220° = 360° Combine like terms

∠QPR = 140° Subtract 220° from both sides

So, ∠QPR=140°.

🔔 What is ∠GJI?

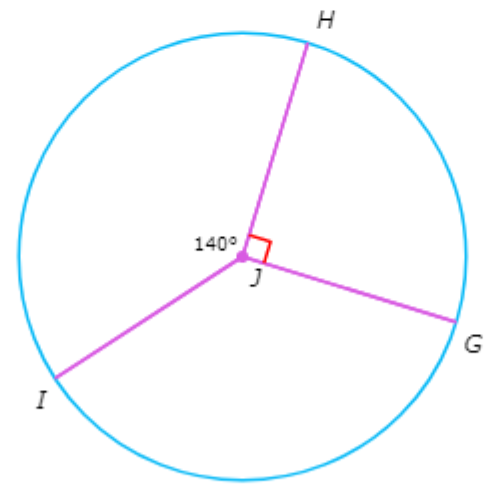
This image has an empty alt attribute; its file name is Untitled-500-%C3%97-500px-1-1.png

∠GJI= _____°

Look at the diagram:

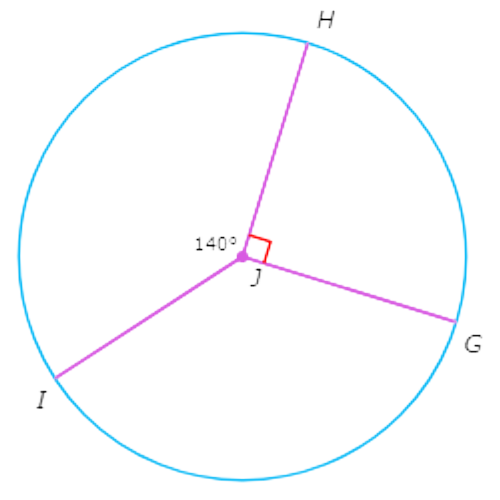
This image has an empty alt attribute; its file name is Untitled__500___500px___1_-removebg-preview-1.png

Write an equation showing that the measures of ∠GJI, ∠HJI and ∠GJH sum to 360°, since they span an entire circle. Then solve for ∠GJI.

∠GJI+∠HJI+∠GJH = 360°

∠GJI+140°+90° = 360° Plug in ∠HJI=140° and ∠GJH=90°

∠GJI+230° = 360° Combine like terms

∠GJI = 130° Subtract 230° from both sides

So, ∠GJI=130°.

Learn with an example

What is ∠GFI ?

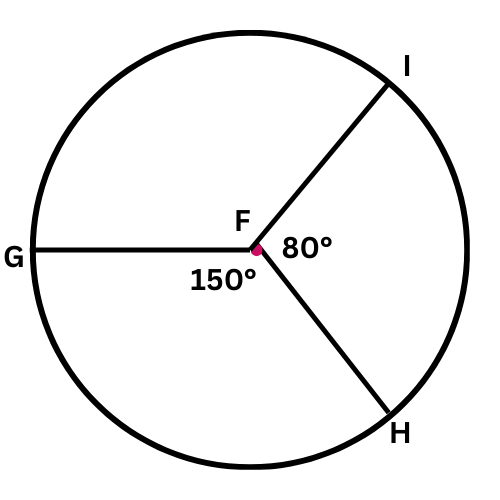
∠GFI = ______°

Look at the diagram:

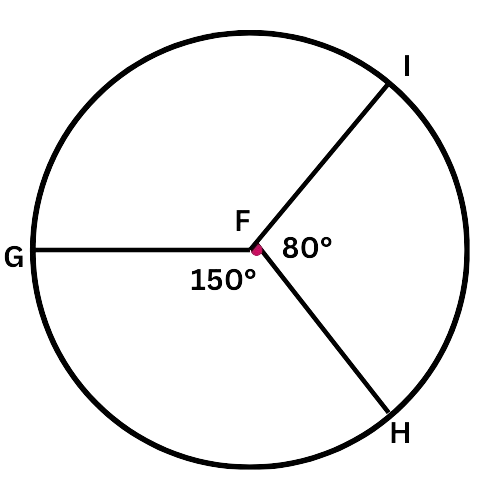
Write an equation showing that the measures of ∠GFH,∠GFI and ∠HFI sum to 360°, since they span an entire circle. Then solve for ∠GFI.

∠GFH + ∠GFI + ∠HFI = 360°

150° + ∠GFI + 80° = 360° Plug in ∠GFH = 150° and  ∠HFI = 80°

∠GFI + 230° = 360° Combine like terms

∠GFI = 130° Subtract 230° from both sides

So, ∠GFI = 130°

What is ∠RQS?

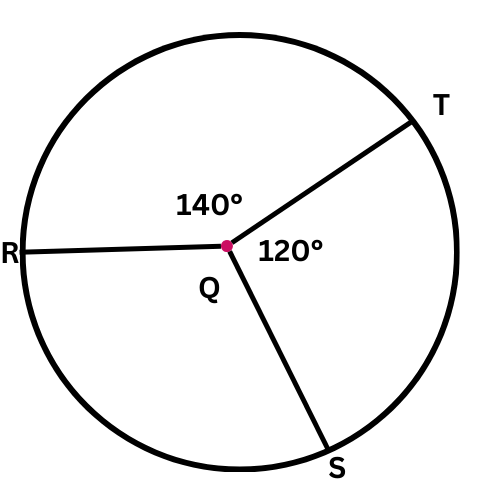
 ∠RQS = _______°

Look at the diagram:

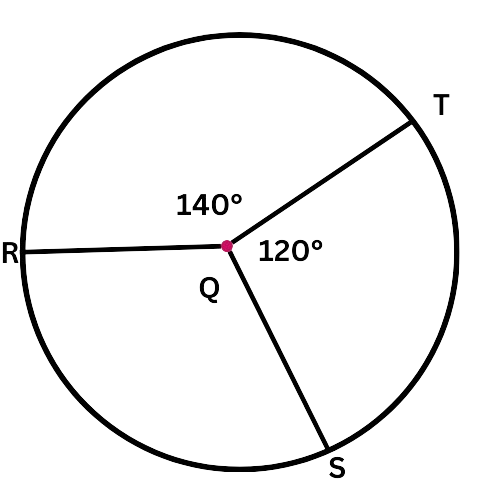
Write an equation showing that the measures of ∠RQS , ∠RQT and ∠SQT sum to 360°, since they span an entire circle. Then solve for ∠RQS.

∠RQS + ∠RQT + ∠SQT = 360°

∠RQS+ 140° + 120° = 360° Plug in ∠RQT = 140° and  ∠SQT = 120°

∠RQS + 260° = 360° Combine like terms

∠RQS = 100° Subtract 260° from both sides

So, ∠RQS = 100°

What is ∠IHJ?

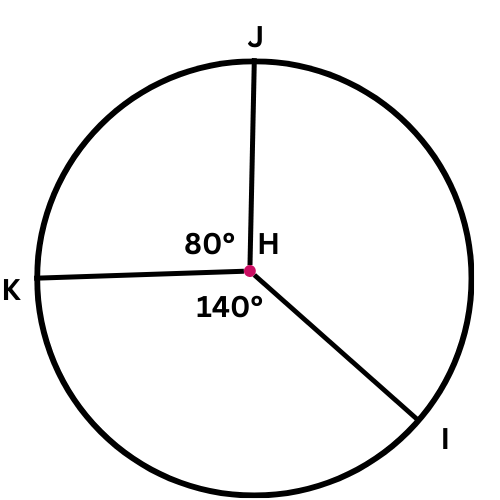
∠IHJ = _______°

Look at the diagram:

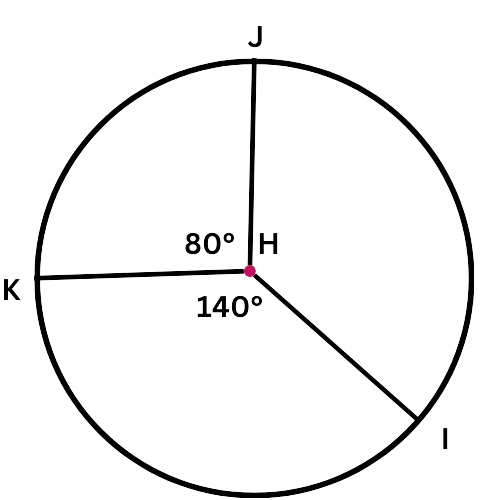
Write an equation showing that the measures of ∠IHK , ∠JHK and ∠IHJ sum to 360°, since they span an entire circle. Then solve for ∠IHJ.

∠IHK + ∠JHK + ∠IHJ= 360°

140° + 80° +∠IHJ = 360° Plug in ∠IHK = 140° and  ∠JHK  = 80°

∠IHJ + 220° = 360° Combine like terms

∠IHJ = 140° Subtract 220° from both sides

So, ∠IHJ= 140°

let’s practice!