Properties of trapeziums
key notes :
Definition of a Trapezium
- A quadrilateral with at least one pair of parallel sides.
- Also called a trapezoid in some regions.
Types of Trapeziums
- Isosceles Trapezium: Non-parallel sides (legs) are equal in length, and base angles are equal.
- Right-Angled Trapezium: Has one pair of right angles (90°).
Basic Properties of a Trapezium
- Has four sides and four angles.
- One pair of opposite sides is parallel (called bases).
- The non-parallel sides are called legs.
- The sum of the interior angles is 360°.
Angle Properties
- Adjacent angles on the same leg are supplementary (sum to 180°).
- In an isosceles trapezium, the base angles are equal.
Diagonal Properties
- A trapezium has two diagonals that may or may not be equal.
- In an isosceles trapezium, diagonals are of equal length.
Midsegment (Median) Theorem
- The midsegment (line joining the midpoints of the non-parallel sides) is parallel to the bases.
- Its length is the average of the two bases:
Midsegment = Base1 + Base2 / 2
Area of a Trapezium
- The area is given by the formula:
Area = 1/2 × ( Base1 + Base2 ) × Height
- Height is the perpendicular distance between the bases.
Symmetry and Reflection
- An isosceles trapezium has one line of symmetry (through the midpoints of the bases).
- A general trapezium may have no lines of symmetry.
Special Cases
- A parallelogram can be seen as a trapezium where both pairs of opposite sides are parallel.
- A rectangle and a square are also special types of trapeziums.
Learn with an example
If UX is not parallel to VW , what is VW?
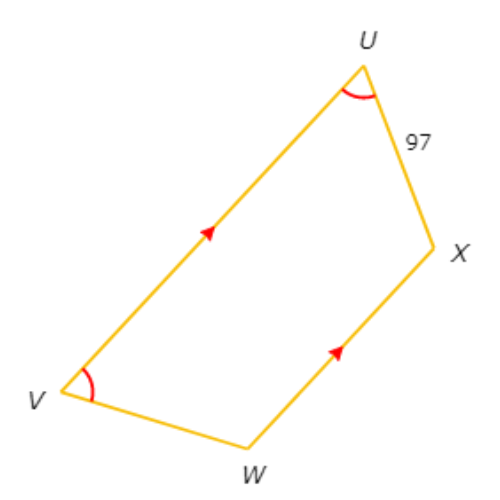
VW= ____
UVWX is a quadrilateral with exactly one pair of parallel sides, UV and WX , and one pair of congruent base angles, ∠U and ∠V.
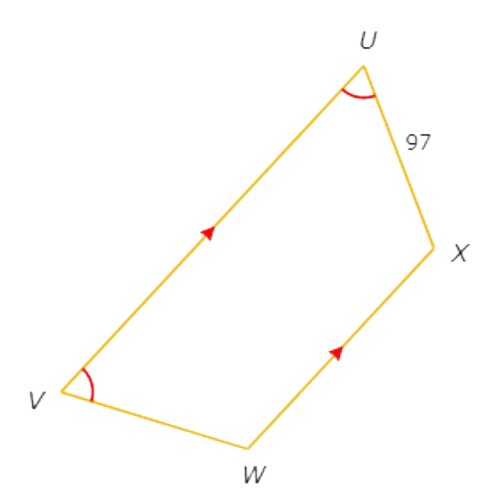
So, UVWX is an isosceles trapezium.
This means the legs are congruent. So, VW=UX=97.
If CD is not parallel to AB , what is CD?
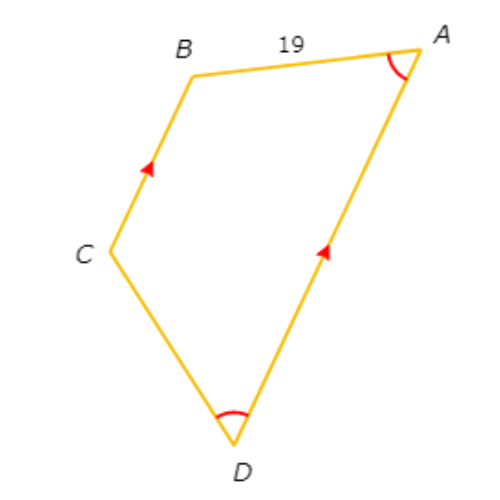
CD =_____
ABCD is a quadrilateral with exactly one pair of parallel sides, AD and BC , and one pair of congruent base angles, ∠D and ∠A.
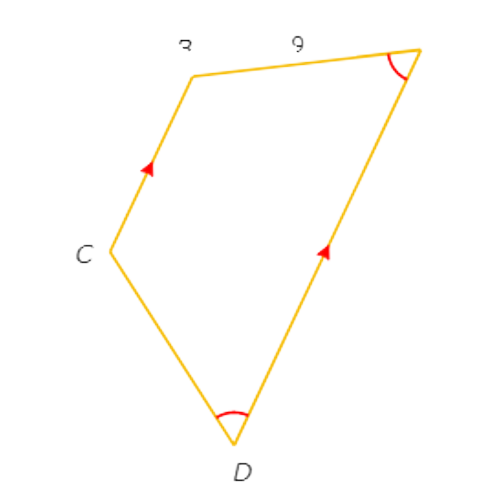
So, ABCD is an isosceles trapezium.
This means the legs are congruent. So, CD=AB=19.
If BE is not parallel to CD , what is BE?
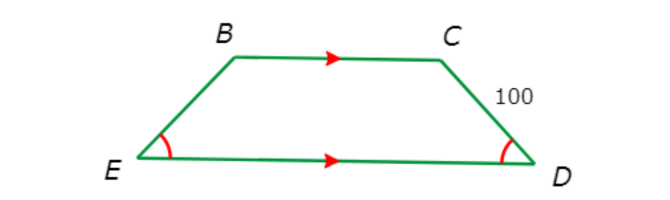
BE = ____
BCDE is a quadrilateral with exactly one pair of parallel sides, BC and DE , and one pair of congruent base angles, ∠E and ∠D.
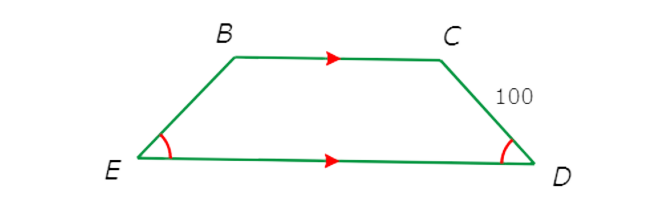
So, BCDE is an isosceles trapezium.
This means the legs are congruent. So, BE=CD=100.
Let’s practice!

